Vector calculus and elementary differential geometry

Green’s integral theorems

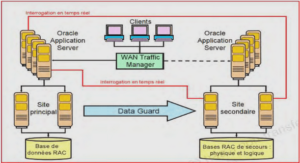
The Green’s integral theorems constitute a generalization of the known integrationby-parts formula of integral calculus to functions with several variables. As is the case with the Green’s function, these theorems are also named after the British mathematician and physicist George Green (1793–1841). They play a crucial role in the development of integral representations and equations for harmonic and scattering problems. As shown in Figure A.16, we consider an open and bounded domain Ω ⊂ R N , that has a regular (strong Lipschitz) boundary Γ = ∂Ω, and where the unit surface normal n points outwards of Ω. 

Divergence integral theorem

The divergence theorem, also known as Gauss’s theorem, is related to the divergence of a vector field. It states that if Ω ⊂ R N is an open and bounded domain with a regular (strong Lipschitz) boundary Γ and with a unit surface normal n pointing outwards of Ω as shown in Figure A.16, then we have for all u ∈ H1 (Ω) and v ∈ H1 (Ω)N that Z Ω div(u v) dx = Z Ω (∇u · v + u div v) dx = Z Γ u v · n dγ. (A.614) By considering u = 1 we obtain the following simpler version of the divergence theorem: Z Ω div v dx = Z Γ v · n dγ. (A.615) The divergence theorem can be proven from the integration-by-parts formula (A.611). In three-dimensional space, in particular, the divergence theorem relates a volume integral over Ω (on the left-hand side) with a surface integral on Γ (on the right-hand side). More adjusted functional spaces for the divergence theorem that still allow to define traces on the boundary can be found in the book of Ned´ elec (2001). 

Curl integral theorem 

The curl theorem, also known as Stokes’ theorem after the Irish mathematician and physicist Sir George Gabriel Stokes (1819–1903), is related with the curl of a vector field and holds in three-dimensional space. There are, though, adaptations for other dimensions.This integral theorem considers an oriented smooth surface Γ ⊂ R 3 that is bounded by a simple, closed, and smooth boundary curve Λ = ∂Γ. The curve Λ has thus a positive orientation, i.e., it is described counterclockwise according to the direction of the unit tangent τ when the unit normal n of the surface Γ points towards the viewer, as shown in Figure A.17, following the right-hand rule. The curl theorem states then for u ∈ H1 (Γ) and v ∈ H1 (Γ)3 that The curl theorem relates thus a surface integral over Γ with a line integral on Λ. We remark that if the surface Γ is closed, then the line integrals on Λ, located on the right-hand side of (A.616) and (A.617), become zero. As with Green’s theorems, more adjusted functional spaces so as to still allow to define traces on the boundary can be also defined for the curl theorem. We refer to the book of Ned´ elec (2001) for further details. 

Other integral theorems

 We can derive also other integral theorems from the previous ones, being particularly useful for this purpose the integration-by-parts formula (A.611). Let Ω be a domain in R N , for N = 2 or 3, whose boundary Γ is regular and whose unit normal points outwards of the domain, as shown in Figure A.16.

Elementary differential geometry

When dealing with trace spaces, we need to work sometimes with differential operators on a regular surface Γ that is defined by a system of local charts, as the one shown in Figure A.15. We are interested herein in a short and elementary introduction to this kind of operators, and for simplicity we will avoid the language of differential forms that is usual in differential geometry, although all the operators which we will describe are of such nature. Let Γ be the regular boundary (e.g., of class C 2 ) of a domain Ω in R N , for N = 2 or 3, which has a unit normal n that points outwards of Ω, as depicted in Figure A.16. For every point x ∈ R N we denote by d(x, Γ) the distance from x to the boundary Γ