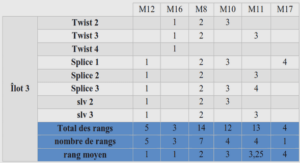
SYSTEM MODELING AND ANALYSIS TOOLS

A wind turbine is mainly composed by rotor, blades, tower and nacelle including gear box and generator, as shown in Figure 1.1; for the floating one, there is an additional floating platform. The principle of energy conversion applied to wind systems is that the kinetic energy of the wind is received by the blades and forces the rotor to rotate. Then, the kinetic energy is transferred into mechanical energy; in the nacelle, the low speed shaft (LSS) rotates with the rotor and is connected to a generator with the high speed shaft (HSS) via a gear box. Then, the mechanical power is transferred into electric energy. , the drive train system and a brief explanation of hydrodynamics of the floating structure. The second part describes the linearized model of the FWT system, for the purpose of control design; a reduced linear model is introduced and compared with FAST nonlinear model. The last part presents the analysis tools, the FWT under interest in this work, and the performance indicators used in this work.-axis is parallel with the chord line (see Figure 1.4-right), pointing to the trailing edge (see Figure 1.4-right). -axis at the blade root; the blade edge-wise moment is caused by the edge-wise force about the x .

Finally, the rotor azimuth angle ψ is defined between the vertical axis and the current position of the blade #1 symmetrical axis (see Figure 1.5). characterizes the efficiency of the conversion of wind energy into mechan- ical energy; such coefficient can be obtained either by real-world experiments or by using accurate simulations. Then, it can be described by different approaches, as look-up tables (Odgaard, Stous- trup, and Kinnaert 2013) (see Figure 1.6) or fitted to a polynomial (Raach et al. 2014) by the following expression versus the TSR λ, for a given blade pitch angle β. It isthen strongly influenced by the wind since λ is a function of V . Then, from (1.1), the power ofIn addition, wind fluctuations induce torque fluctuations, increasing the fatigue loads on the drive shaft and also affecting the quality of the produced power produced. So, there is a real interest in designing variable speed wind turbines, to adapt their rotation speeds to wind variations in order to obtain the most appropriate C value and therefore to optimize the power output in different operating regions (for definition of regions, see previous chapter). The aerodynamic torque developed by the turbine blades is defined by (Guenoune et al. 2017; Huang, F. Li, and Jin 2015)

The purpose of the drive train is to transmit wind power and mechanical torque from the turbine to the electric generator (Burton, Sharpe, and Jenkins 2001). There are different types of drive train models, depending on the number of the mass, such as six-mass, three-mass and two-mass models (Muyeen et al. 2007). Among those models, the two-mass model has been widely used in the literature (Novak, Jovik, and Schmidtbauer 1994; Beltran, Ahmed-Ali, and Benbouzid 2008; Abo- Khalil et al. 2019). The complexity of the two-mass model is reduced, but this mdoel is sufficient for characterizing the dynamics of the drive train (McFadden and Basu 2016). representing the inertia of the mechanical part (blades,tower and hub) and electrical part (generator rotor) respectively, a low speed shaft (LSS) modeled by a torsional spring and a torsional damper, a rigid high speed shaft (HSS), and finally a gear box Different from the fixed-bottom wind turbine, due to the presence of a floating platform, additional 6 DOFs (platform horizontal surge, horizontal sway, vertical heave translation and platform roll, pitch, yaw rotation – Figure 1.2) must be considered for the floating wind turbines. These 6 DOFs are infected by the hydrodynamics forces caused by waves, mooring system, and by the aerodynamic forces caused by the wind. Of course, all these factors have a great influence on translations and rotations of the platform. Additionally, in the offshore environment, the dynamics of the platform are highly nonlinear and coupled with the aerodynamics of the turbine system; it is clearly a very complex task to model the hydrodynamics in control design context (Jason M Jonkman, M. L. Buhl Jr, et al. 2005; Y. Shi et al. 2017; Cho 2020). Therefore, specific models for FWT are required in a reduced scale and will be introduced in the next section.