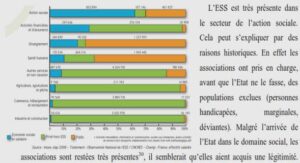
- Saturday
- April 26th, 2025
- Ajouter un cours

Types of input data We have benefited of several types of data in order to obtain the thermal emission of a variety Earth-like planets. First, we have used high-resolution thermal emission data of the Earth (Section 3.1), the data are...

Aspects épistémologiques des figures et du raisonnement pour l’entrée dans la géométrie théorique Figures géométriques Figure géométrique et visualisation non iconique Dans le programme scolaire du cycle 1, on parle de manipuler, reproduire, dessiner, identifier et décrire des « formes...

An improper estimator with optimal excess risk in misspecified density estimation and logistic regression Abstract. We introduce a procedure for predictive conditional density estimation under logarithmic loss, which we call SMP (Sample Minmax Predictor). This estimator minimizes a new general...

Wave propagation Waves, as summarized in the insightful review by Keller (1979), are disturbances that propagate through space and time, usually by transference of energy. Propagation is the process of travel or movement from one place to another. Thus wave...
FULL-PLANE IMPEDANCE LAPLACE PROBLEM In this appendix we study the perturbed full-plane or free-plane impedance Laplace problem, also known as the exterior impedance Laplace problem in 2D, using integral equation techniques and the boundary element method. We consider the problem...
HARBOR RESONANCES IN COASTAL ENGINEERING In this chapter we consider the application of the half-plane Helmholtz problem described in Chapter III to the computation of harbor resonances in coastal engineering. We consider the problem of computing resonances for the Helmholtz...

Vector calculus and elementary differential geometry Green’s integral theorems The Green’s integral theorems constitute a generalization of the known integrationby-parts formula of integral calculus to functions with several variables. As is the case with the Green’s function, these theorems are...
Relaxed lifting of triangular sets Notations Throughout this chapter, we use the notions and notations of Chapter 1, Section 1.1. In particular, we use the ring of p-adics Rp with its assumption on the length function λ and its complexity...

MATHEMATICAL AND PHYSICAL BACKGROUND A short survey of the mathematical and physical background of the thesis is presented in this appendix. The most important aspects are discussed and several references are given for each topic. It is thus intended as...