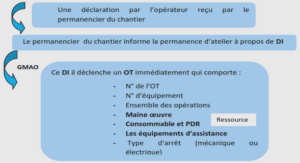
On some boundary value problems at resonance 

In this chapter we collect some interesting results for various classes of second boundary value problems at resonance. More precisely, we will summarize basic results in the literature related, and present the main ideas of some researches in resonant boundary value problems. we omit the corresponding proofs. 

A Second order m-point boundary value problem at resonance

Gupta in [25], used Mawhin coincidence degree theory, to investigate the existence of solutions for the following two boundary value problem at resonance, (P1) ( u 00(t) = f(t; u(t); u0 (t)) + e (t); t 2 (0; 1); u(0) = 0; u0 (1) = u 0 ()   (P2) 8 < : u 00(t) = f(t; u(t); u0 (t)) + e (t); t 2 (0; 1); u(0) = 0; u0 (1) = mP

On multipoint boundary value problem at resonance

In [34], Liu and Yu considered the following second-order ordinary di§erential equation u 00(t) = f(t; u(t); u0 (t)) + e (t); t 2 (0; 1); (3.1) subject to one of the following boundary value conditions: u(0) = mX