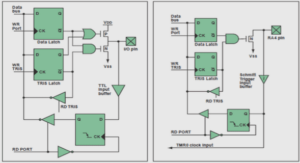
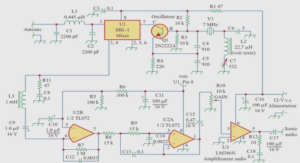
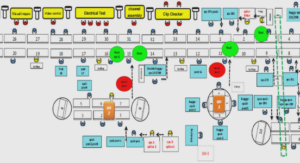
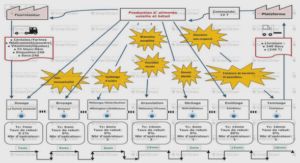
NASA SRB data

Time series analysis Two examples of the annual time series of Earth’s outgoing mid-infrared radiation are plotted in Figure 4.2. The high frequency variability corresponds to the emitted mid-infrared flux due to Earth’s rotation superimposed to the seasonal variation during the year. It is readily observable from the figure that the amplitude of the rotational variability is larger for an observer in the equatorial plane (black) and decreases toward more poleward views (violet, magenta). On the contrary, because the obliquity of the Earth is about 23.44◦ and then the variation of the annual insolation is larger at higher latitudes, the amplitude of the seasonal variability increases for the polar geometries and decreases towards an equatorial view. As it is expected, the seasonal variation of the northern latitude time series is opposite to the southern one due to the seasonal cycle of solar insolation. For observers over the Northern Hemisphere, the time series reach a maximum during the boreal summer near the beginning of August (orbital period fraction)(opf)∼ 0.6). The equatorial view follows the same pattern, showing a “northern-biased” behavior of the planet. As we have explained in the previous section, this is due to the existence of large landmasses in the Northern Hemisphere that emit more infrared radiation than the oceans and make the northern summer hotter. In the time series, this effect is accentuated by the planetary view. Table 4.1 shows the amplitude values of the seasonal variability of the globally integrated mid-infrared flux for the years of 1987 and 2001 and for five viewing geometries: 0◦ (Equator), 45◦N, 45◦S, 90◦N (North Pole) and 90◦S (South Pole). The amplitude is given in percentage change over the annual mean value. In order to conduct a seasonal study of the mid-infrared Earth emission, four representative months (January, April, July and October) were selected per year. For the sake of simplicity, we present in particular the results of the year 2001, however the rest of the data set give similar results. For comparison, we give the values taken by the observers placed in the opposition and in the conjunction planes (in parenthesis), because they have the same view of the planet at different local hours. We can see that the orbital amplitude variation of a northern latitude is twice the value of the southern equivalent latitude. The seasonal variability dominates the signal in most latitudes except for the equator, where seasonal and rotational variability are similar. It is notable that whereas the mean temperature varies with the season, the rotational variability depends strongly on the local hour (Section 2.2). This effect can be seen in the black graphs of the two bottom charts of Figure 4.2, each graph correspond to two observers at the equator plane at opposite sides of the planet. The observer placed at opposition the 1st of January (opf=0) sees the planet at midnight, whereas the observer placed at conjunction sees the planet at midday. In summer (opf∼ 0.5), the planetary view is the opposite, the observer at opposition sees the planet at midday and the observer at conjunction sees the planet at midnight. Mid-latitude and equatorial viewing geometries show a pronounced and synchronized rotational variability (up to 8% in change), whereas the polar light curves present almost no variations.

Periodicities

The duration of the statistically significant peaks in the autocorrelated time series can give us an estimation of the lifetime of the cloud structures, typically of around one week for Earth clouds (Pall´e et al., 2008). In the outgoing mid-infrared radiation flux, a 24 hr rotation period is clearly shown, a value close to the true rotation period. This rotational signature has two origins. First, some large regions exhibit systematically high or low brightness temperatures. This is the case for Indonesian and Sahara areas, as the former is one of the most humid and cloudy regions on the planet, whereas the latter is warmer and drier than Earth’s average. Therefore, the two regions appear as fixed cold and hot features respectively, even on averaged outgoing flux maps, as we can see in Figure 4.1(c) and (d) where the TOA emission is averaged over a month and in Figure 4.1(e) and (f) where it is averaged over a year. A smaller effect comes from the diurnal cycle (the change of brightness temperature between day and night in a region), which is negligible in most locations, because of humidity, clouds, or ocean thermal inertia, but noticeable in some dry continental areas as we discuss in the next section. In fact, it is the diurnal cycle of the dry lands, which makes possible the detection of the rotation period for the case of the North-polar view during the more stable seasons (winter and summer), as it is shown in Section 2.2. Although it is always the same fraction of the planet (the whole northern hemisphere) in the field of view, the change in temperature along the day in the dry continental areas causes the rotational modulation, as it is shown in Figure 4.3 (violet solid line). However, an observer looking at the South Pole does not detect a significant rotational variability, Figure 4.3 (violet dash-dotted line), not even for the austral summer when the effect of clouds would be minimized. In the Southern Hemisphere, the distribution of land is largely dominated by oceans whose high thermal inertia make diurnal temperature variability negligible. 

Average Rotation Light Curves 

Once the rotation period is identified, the observer can produce a typical rotation light curve by folding the time series obtained during weeks or months over the rotation period. This process averages out random cloud variability. It is clearly seen on the maps of Figures 4.1(c)–(f), where the clouds disappear for longer average times whereas the strongest features mentioned on the previous section prevail. Then the observer can plot this average rotation light curve as a function of an arbitrary longitude (in our case the longitude that we have chosen is the conventional geographic longitude for commodity). The shape of the light curve can then reveal brighter/fainter areas distributed in longitude. For instance, observers over a latitude of 30◦N would note that the brightest and faintest point of the light curve occur when the longitude 0◦ (Sahara) or 135◦ (Indonesia) are respectively centered on his view. The results are represented in Figure 4.4 and Figure 4.5. Figure 4.4 represents the rotational variability respect to the local hour for several latitudes (in column) at the months of January (top row) and July (bottom row). Each graph corresponds to four observers initially placed at the four astronomical longitudes (meridional planes) O, C, WQ, EQ, previously defined, at the same latitude (at the top of each chart). Each of these four meridional planes correspond to a certain local hour that changes with the orbital movement of the planet. With this information, we can compare the temperature evolution for a given planetary region along the day (diurnal variability). The figure shows that the longitudes that reach the maximum of temperature, have also the largest variation along the day; whereas the 76 4. NASA SRB data Figure 4.6 TOA–LW infrared emission flux of the Northern and Southern hemispheres. Total emission (top) of the Northern Hemisphere (black) and Southern Hemisphere (red). Latitude bands (bottom) of 90◦N–60◦N (blue), 60◦N–30◦N (green), 30◦N–0 ◦ (orange), 90◦S–60◦S (black), 60◦S–30◦S (magenta) and 30◦S–0 ◦ (red). longitudes where the minimum is attained, show a little variation. For the cases where the observers are placed over the poles, the planetary view does not change with time so the local hour of the graph is taken just as reference of the observation time (Section 2.1). For the North Polar view, not only during summer but also during winter, the minima occurs when it is 0 UT (0 hr at 0◦ longitude or 12 hr at 180◦ longitude) and the maxima when it is 12 UT (0 hr at 180◦ longitude or 12 hr at 0◦ longitude), which illustrates the diurnal cycle effect previously mentioned. These hours coincide respectively to midnight and midday in the Sahara desert. The greatest influence of this region is noticed at the chart of 30◦N latitude when the Sahara-Arabian region (15◦W-50◦E) is in the center of the planetary disk. The rotational amplitude is also influenced by the seasonal cycle, as we can see in the flux difference between January and July. Either in summer or winter the diurnal variability reaches the 2% at low 4.4. Average Rotation Light Curves 77 Figure 4.7 TOA–LW infrared emission flux of some continental regions in the Northern Hemisphere. In the 60◦N–30◦N latitude band (top): Europe (red), Asia (blue), and USCanada (green). In the 30◦N–0 ◦ latitude band (bottom), Sahara-Arabian (red), Indonesian (blue), and Caribbean-Mexico area (green). latitudes, although the main contribution to the flux is due to the solar insolation along the year (seasonal cycle) and to the rotation variability. For Polar views, the amplitude is only due to the diurnal variability. Figure 4.5 represents the rotational variability for observers placed at 0◦ longitude and different latitudes. It is important to note the temperature evolution with time for each geometry, implying a seasonal behavior. The equator shows a warm stable temperature during the year, whereas the summers of each hemisphere differ being the northern summer hotter, as it is shown in Figure 4.4. The maximum and minimum regions do not change with the seasons, except for April and October when the presence of the clouds can mask the signal. In order to check the possible source of the diurnal variation on the Northern Hemisphere, we have made a further analysis of the emitted flux by geographic 78 4. NASA SRB data Figure 4.8 Time series for a North Polar observer (black) and the contribution of each latitude band to the signal. 90◦N–60◦N latitude band (blue), 60◦N–30◦N (green) and 30◦N–0 ◦ (orange). latitude bands. Figure 4.6 shows the mid-infrared emission of the Earth along one orbital period. Each hemisphere is divided in three latitude bands of flux: 90◦N– 60◦N (blue), 60◦N–30◦N (green), 30◦N–0◦ (orange), 90◦S–60◦S (black), 60◦S–30◦S (magenta), 30◦S–0◦ (red). We can see that the Northern Hemisphere exhibits a larger seasonal variation than the Southern Hemisphere and this variation is more important for mid-latitudes. As it was previously mentioned, the greater diurnal variability comes from the latitudinal band 30◦N–0◦ , also in this band, there is a decrease in flux during summer. This is due to the migration of the Intertropical Convergence Zone to these latitudes, which produces large bands of humidity and clouds with low brightness temperatures. As expected, we can observe that the Southern Hemisphere is in general colder than the North Hemisphere, and this difference is particularly remarkable between the South and the North poles. 

Phase Variation 

While Earth’s visible flux received by a remote observer is modulated by the changing phase of the planet, the Earth does not present significant phases when the integrated infrared flux is observed: the emission from the nightside contributes nearly as much as the emission from the dayside (Selsis, 2004). As we have previously explained, the diurnal variability (1%-2%) is in general lower than the rotational (1%-8%) and the seasonal variability (5%-20%). This is shown in Figure 4.2 (middle and bottom), which represent opposite observers and then opposite visible phases of the planet. An observer at opposition (O) sees the winter midnight, the observer at conjunction (C) sees the winter noon which shows a similar average temperature but a larger rotational variation. Figure 4.4 shows this difference between observers at O, C, WQ and EQ. The effect is amplified for warm areas at low latitudes, as the Sahara desert, as they have the larger contribution to the signal and it becomes more important for observers also at low latitudes, as the area pass by the center of the planetary disk. The equatorial observers of Fig. 4.2 (black) are represented by the graphs at 0 hr and 12 hrs at the central chart (0◦ ), although we can see the difference of phase angle in the signal, the rotational and seasonal contributions dominate. Continental surfaces and the boundary layer above them (roughly the first 1500 m of the atmosphere) experience a drop of temperature between the day and night. This diurnal cycle is insignificant above the ocean (due to the high thermal inertia of water), hence over ∼ 70% of the Earth surface. Day–night brightness temperature variations affect the outgoing thermal emission only in the 8–12 µm atmospheric window and above dry continents. This happens either over very cold regions which in this case do not contribute much to the global emission, or over deserts which represent a small fraction of the Earth surface. In addition, the diurnal cycle is much less pronounced above the boundary layer, at altitudes where most of the thermal emission is emitted to space. This is the reason why phasecorrelated variations of Earth brightness temperature are negligible compared with seasonal changes. Even for the observing latitudes in the 30◦N–0◦ range, in which the Sahara diurnal cycle appears in the modulation, the winter midday is colder than the summer midnight as it is seen in Figure 4.4.