Hydrocarbon migration modeling in basin simulators

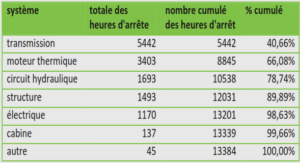
Hydrocarbon migration modeling in basin simulators

Introduction

This paper addresses the modeling of the two main processes that occur at geological time-scales in sedimentary basins, namely secondary and tertiary hydrocarbon migration. Before elaborating on the scope of our study, let us briefly recall the nature of these phenomena in the context of the limited physical properties taken into account in basin modeling. Secondary migration is the movement of hydrocarbons along a carrier bed from the source rock to the trap. As shown by Schowalter (1979) and England et al. (1987), it can be accounted for by three physical mechanisms. The first and main driving process is buoyancy. « When two immiscible fluids (hydrocarbon and water) occur in a rock, a buoyant force is created owing to the density difference between the hydrocarbon phase and the water phase. The greater the density difference, the greater the buoyant force for a given length hydrocarbon column (always measured vertically) » (Schowalter, 1979, p. 10). The second process is hydrodynamics. It adds a force that may be in any direction, depending on the nature of the flow involved (England et al., 1987). Indeed, the buoyant force can be reduced or increased when a hydrodynamic condition exists in the subsurface. However, the effects of hydrodynamics are not always of the utmost importance (Carruthers, 1998). The third process is capillary pressure. This is in fact a resistance effect which controls the hydrocarbon trajectories. The factors that determine its magnitude are the radius of the pore throat of the rock, the hydrocarbon-water interfacial tension and wettability (Schowalter, 1979).  The combination of these three processes leads to the ascent of hydrocarbons through the carrier beds until the capillary pressure is sufficient to offset the effects of the difference of densities and hydrodynamics. Note that we have neglected compaction as a driving force for secondary migration as this is commonly assumed. Tertiary migration is the leakage of hydrocarbons from traps. It is attributed to capillary leakage, hydraulic leakage and molecular diffusion (Sylta, 2004). Caprock leakage is possible when the driving processes (buoyancy, pressure gradients and molecular diffusion) exceed the resistant factors (capillary entry pressure or permeability) of the confining barrier (Thomas and Clouse, 1995; Burrus, 1997). In a normal pressure accumulation, a caprock reaches its maximum seal capacity when the pressure generated by the hydrocarbon column is equivalent to the capillary entry pressure of the barrier. For an accumulation in overpressure (i.e., the difference between the fluid pressure and the hydrostatic pressure), the direction and the magnitude of fluid circulations are controlled by the global pressure field and the buoyancy generated by the hydrocarbon column is not the main force. The rate of leakage is then controlled by the permeability, the fluid viscosity and the pressure gradient (Watts, 1987; Schlomer and Krooss, 1997). Two major techniques are commonly used to model secondary and tertiary hydrocarbon migration: Darcy flow and invasion percolation. These approaches differ from each other in many ways, most notably in the physical modeling, the methods of resolution, and the type of results obtained. This paper aims to summarize, compare and illustrate these two techniques through particular case studies. Its purpose is to highlight the capabilities of the different methods developed and to underline the advantages and drawbacks of each. Although it does not claim to add any insight into the physics and mechanics of hydrocarbon migration itself, we believe that such a comparison can help the practitioners who use migration modeling. This paper is outlined as follows. First, we describe the Darcy approach, its physical principles, some standard numerical methods of resolution, and their limitations in Section 2. Section 3 is devoted to the invasion percolation approach, its algorithm and limitations. Then, we recapitulate the characteristics of each approach in Section 4. Finally, we illustrate their main differences through examples in Section 5. 

Darcy approach 

Darcy flow models assume that hydrocarbon displacement honors the Darcy law extended to multiphase fluids (Bear, 1972; Marle, 1972). Migration is driven by buoyancy, fluid pressure field and capillary pressure. Darcy migration is simulated by solving partial differential equations and the numerical treatment of the full set of equations is generally considered computationally costly and quite complicated (Schneider, 2003).

Physical principles 

Based on the results of experiments on the water flow through beds of sand, Darcy (1856) formulated the law P K U = − ∇ µ where, U is the Darcy velocity (m/s), K is the permeability of the rock (m2), P is the pressure (Pa), µ is the viscosity of the Newtonian fluid (Pa.s). From the theoretical viewpoint, it has been proved that Darcy’s law is not a constitutive law but a simplified form of the homogenized Navier-Stokes model (Hubbert, 1956; Irmay, 1958; Bear, 1972; Whitaker, 1986). The coefficient µ K is a viscous term due to friction at the solid-fluid interface. Moreover, in order to generalize Darcy’s law to multiphase flow, the simplest approach is to assume that each fluid phase maintains a network of passages; the wetting fluid in the larger pores, with friction between fluid and solid (Bear, 1972). In addition to the three main processes already mentioned (buoyancy, capillary forces and pressure gradient), the extension of Darcy’s law to multiphase flow in porous media uses the concept of relative permeability. For two phases, this permeability correction term reflects the permeability reduction of a fluid flow caused by the presence of the second fluid in the porous medium (Guérillot and Kalaydjian, 1988). Then, the extended Darcy law reads 

Numerical modeling 

Darcy model is classically coupled with a pressure-compaction model. This means not only that hydrocarbon migration depends on the pressure-compaction computation, but also that the pressurecompaction is influenced by the migration computation. Basin modeling simulators usually simultaneously solve the multiphase Darcy law, the mass-conservation equations for solid and fluids, and a compaction law. To solve this set of equations, finite difference, finite element or finite volume methods are used for the spatial discretization. Various time-schemes are also employed for the transport equations: the Impes with an implicit treatment for the pressure computation and an explicit one for all other unknowns; the Impims based on an implicit treatment for all the unknowns. These two time strategies solve sequentially in two separate stages the pressure-compaction problem and the hydrocarbon transport equations. On the contrary, with the Fully Implicit scheme, we have to solve a coupled system of non-linear equations for pressure and hydrocarbon saturation. All of these schemes have distinct advantages and limitations (Wolf et al., 2011), but in all the cases, performing a simulation with a complete Darcy model is expensive in computing time. Indeed, to treat the non-linearity of the equations, a classical Newtonian scheme is used. The convergence of this scheme may be a delicate issue in some cases and may cause the time-step to decrease, particularly when a huge amount of hydrocarbon migrates rapidly. At each Newton iteration, solving the linear system represents a huge CPU-time-consuming part of the simulation (Willien et al., 2009). Furthermore, pressure dependent flow can significantly increase the computing time, especially in highly permeable layers. The management of computing time steps depends also on the strong heterogeneities of the fluid properties. Nevertheless, parallel techniques and specific preconditioners can improve the computing time for the Darcy approach (Requena et al., 2005). 

Limitations

 It is a classical fact that Darcy’s law can be considered as valid only for slow Newtonian flows, i.e., for Reynolds numbers between 1 and 10 (Bear, 1972; Burrus, 1997). It is also well-known that Darcy’s law breaks down in extremely fine-grained clayey soils. The multiphase nature of the flow is likely to further restrict the validity of the Darcy model. Indeed, in two-phase systems, when the capillary term becomes important at « relatively low pressures, no continuous pathway through the rock is possible, and no flow will occur […] this type of non-linear behaviour is obviously inconsistent with Darcy’s law » (England et al., 1987, p. 335). In an attempt to reduce the computation cost of Darcy approach, it is often suggested to use large cells. This requires permeability, relative permeability and capillary properties to be upscaled on a lowresolution numerical mesh. However, “the use of constant oil saturation in each computing cell results in too large average saturations being modelled when the vertical migration pathway has to overcome tight zones” (Sylta, 2004, chap. 10, p. 13). Due to this low resolution, Darcy approach tends to overestimate migration losses during secondary migration.