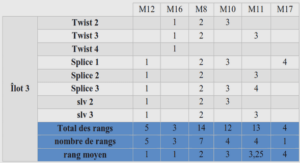
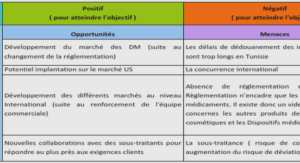
Hybrid experimental-numerical characterization of the effect of strain rate on fracture

includes notched as well as uniaxial tension specimens. Local displacements and surface strain fields are measured optically in all experiments using Digital Image Correlation. Constitutive equations derived from the Mechanical Threshold Stress theory are proposed to describe the rate-dependent behavior as well as plastic anisotropy of the sheet material. Detailed Finite Element simulations of all experiments reveal that the model accurately predicts experimental results, including force displacement curves and local surface strain evolution. In particular, the behavior at large strain, beyond the onset of necking, is well predicted. Stress and strain histories where fracture initiates are also obtained from the simulations in order to characterize the dependence of the material ductility to both strain rate and stress state. If the fracture strain is higher at high strain rate in all experiments, results show that the effect of strain rate on ductility cannot be considered independently from the state of stress. The plastic deformation behavior of AHSS steels under uniaxial tension has been extensively studied during the last decade, indicating that this class of material experiences positive strain rate sensitivity (e.g. Khan et al., 2012, [91]). Experiments in experiments (100 – 1,000s-1) are usually performed in Split Hokinson bar systems (e.g. Van Slycken et al., 2007, [182]; Dunand et al., 2013, [47]) or direct impact setups (e.g. He et al., 2012, [76]). A key advantage of uniaxial tension experiments is that they give a direct access to the material response at small strain levels, and especially to the dependence of flow stress to strain rate. Indeed, force and local displacement measurements are straightforwardly related to the stress and strain within the range of uniform elongation. Used in conjunction with optical strain measurement methods, it also permits to evaluate the effect of strain rate on plastic anisotropy of AHSS sheets (e.g. Huh et al., 2013, [83]). With the exception of a few experimental results reported for shear loading conditions (e.g. Rusinek & Klepaczko., 2001, [155]; Peirs et al., 2011, [147]), virtually no data can be found in the literature describing the multi-axial behavior of AHSS sheets at high rates of strain.

The effect of strain rate on the ductility of AHSS has comparatively been less evaluated. Most experimental investigations are limited to characterizing uniaxial tension parameters such as the ultimate elongation (e.g. Olivier et al., 2007, [141]; Huh et al, 2008, [82]), thereby putting aside the effect of stress state on ductile fracture. Results presented do not permit to draw a clear trend in the dependence of ultimate elongation to strain rate for AHSS steels. Kim et al. (2013, [94]) measured increasing ultimate elongations for increasing strain rates (ranging from 0.1s-1 to 200s-1) in case of a DP780 and a TRIP780 steel. Similarly an elongation twice higher at 1000s-1 than at 0.001s-1 is found for a TRIP steel by Verleysen et al. (2011, [185]). On the other hand, is emphasized that the ultimate elongation in a uniaxial tension experiment is a structural characteristic and not an intrinsic material property. It depends strongly on the specimen geometry (Verleysen et al., 2008, [184]; Sun et al., 2012, [168]) and is thus a questionable indicator of material ductility. A somewhat more reliable evaluation of the fracture strain in uniaxial tension experiments may be obtained by measuring the reduction of the cross-section area on fractured specimens and assuming a uniform strain distribution through the sheet thickness. Based on this Reduction of Area method, Kim et al. (2013, [94]) report a decreasing fracture strain for increasing strain rates in case of a TRIP780 steel, and a non-monotonic dependence of ductility to strain rate for DP780 steel.

In most fracture experiments on sheet materials, however, the localization of plastic deformation through necking cannot be avoided and represents a major difficulty in the accurate characterization of the material state at fracture. After necking, the stress and strain fields within the specimen gage section become non-uniform and of three- dimensional nature (stresses in the thickness direction develop). Consequently, the stress history prior to fracture can no longer be estimated from the force history measurements using simple analytical formulas: stress and strain histories prior to fracture need to be determined in a hybrid experimental-numerical approach (Dunand & Mohr, 2010, [48]). In other words, a detailed finite element analysis of the experiment is required to identify the stress and strain fields up to the onset of fracture.

An extremely wide variety of constitutive equations describing the rate- and temperature-dependent plastic behavior of metals have been proposed. The goal of constitutive equations is to relate the flow stress to strain, strain rate and temperature (and possibly other internal variables) in order to describe the basic mechanisms of strain hardening, strain rate sensitivity and thermal softening (and possibly damage, phase transformation, texture evolution…). Rate-dependent plasticity models can be categorized into two main branches. On the one hand phenomenological models are based on the separation of the effects of strain, strain rate and temperature (e.g. Johnson and Cook, 1983, [88]; Cowper and Symonds, 1952, [39]). Strain hardening, strain rate sensitivity and thermal softening mechanisms are described by means of basic functions, depending respectively on strain, strain rate and temperature only, which may be arbitrarily combined in additive or multiplicative manners to build an ad-hoc model. A partial review of phenomenological basic functions can be found in Sung et al. (2010, [169]). Integrated phenomenological models in which variables are not separated have also been proposed. They allow for description of more complex mechanisms: temperature-dependent strain hardening (e.g. Sung et al., 2010, [169]), rate-dependent strain hardening (e.g. Khan et al., 2004, [93])… On the other hand, attempts have been made to incorporate a description of the mechanisms of plasticity into physics-based constitutive models (e.g. Zerilli and Armstrong, 1987, [195]; Follansbee and Kocks, 1988, [58]; Voyiadjis and Abed, 2005, [187]; Rusinek et al., 2007, [157]). Most physics-based models make use of the theory of thermally activated plastic deformation to relate flow stress, strain rate and temperature (Conrad, 1964, [38]; Kocks et al., 1975, [95]). Additional internal variables can also be introduced in the constitutive equations to account for history effects (Bodner and Partom, 1975, [20]; Durrenberger et al., 2008, [52]). Comparisons between phenomenological and physics-based models against experimental data tend to show that the latter offer better predictive capabilities when a wide range of strain rates and/or temperatures is considered (Liang and Khan, 1999, [111]; Abed and Makarem, 2012, [2]; Kajberg and Sundin, 2013, [90]).