Exaltation de l’onde évanescente leguide d’onde résonnant

The use of the dipole force in an evanescent wave to reflect atoms was proposed in [1], and has received a great deal of experimental attention [2-12]. This is because such a device is a very promising way to make an atomic mirror, and our experience in optics leads us to expect that good mirrors will be important tools for atom optics. To be really useful it is important that the atomic mirror be ‘coherent’, i.e. that it preserve the coherence of the incident wave. Thus, random processes such as spontaneous emission must be avoided. One can show that, for a tixed incident velocity, the minimum probability of spontaneous emission during the bounce is inversely proportion al to the laser intensity creating the evanescent wave [6,10]. In alI the experiments of [2-6], the spontaneous emission probability was equal to or greater than about 10% per atom per reflection. It is therefore important to increase the intensity of evanescent waves to obtain coherent atomic mirrors. A solution for reaching higher intensities is to enhance the evanescent wave using a passive resonant structure. Atomic mirrors based on this idea have been demonstrated. making use of both surface plasmons [7-9] and of a dielectric waveguide structure [10-13].

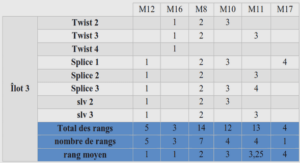
The waveguide used in [10], was described in a previous paper [14J. The principle of the enhancement was explained, and we showed that the main features of the structure could be qualitatively understood using an analogy with a Fabry-Perot cavity. We also introduced a method to evaluate the enhancement, based on the analysis of the reftected light. The treatment of the experimental data yielded a value of 130 for the enhancement factor, the ratio of the effective intensity €ocE2/2 of the evanescent wave at the interface with and without the waveguide. In this tirst system, the influence of the losses was negligible. In this paper, we describe an improved structure with an enhancement factor of 1650, a gain of more than one order of magnitude. With this structure we were able to reftect atoms with a spontaneous emission probability below 1 of this highly resonant system requires a more detailed analysis than in the previous case. In particular, the losses have to be accounted for to calculate the enhancement. We will also use a Lorentzian approximation to derive sorne simple analytical expressions for the transmission and reftection coefficients of the structure as a function of the wavelength [15]. This provides a simple understanding of the influence of the various parameters of the structure and a straightforward way to estîmate the enhÎii1cement, using the quantities characterizing the reflectivity curve. We have applied this method to our device placed in the conditions of the atomic mirror experiments (i.e. inside a UHV chamber). We measure the intensity of the light reftected by the structure as a function of the wavelength, and use the quantities characterizing this reftectivity curve to calculate the enhancement factor. This remote measurement technique pennits easy in situ characterization of the resonance. It has enabled us to observe the evolution of the structure with time, a phenomenon that we attribute to a reversible pollution of the prism surface by rubidium atoms.

In section 2, we present the detailed analysis of the enhancement of evanescent waves using a dielectric waveguide structure. We describe in section 2.1 the structure of the multilayeredsystem, and recall the simple anal ogy with a Fabry-Perot cavity which allows a qualitative understanding. We then introduce the notation used throughout the paper, and recall the results of the exact plane-wave treatment based on the Fresnel coefficients (section 2.2). In sections 2.3 and 2A, we discuss the behaviour of the device in the ‘low-coupling’ regime, analogous to a high reftectivity input coupler in a Fabry-Perot, both without and with losses in the waveguide layer. Two different regimes appear, depending on the value of the losses with respect to the coupling. In section 2.5, we use the Lorentzian approxÎmation to derive sorne simplified expressions for the transmission and reftection coefficients of the structure around resonance. Using these results, we show that the enhancement can be easily obtained through the analysis of the reftected Iight.