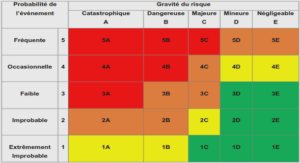
Complémentarité des lois de contrôle

Predictions

Walking task

In accordance with our previous study (François et al., under review), manipulating GOFR via Eye Height should give rise specific speed adjustments. More particularly, participants should decrease their walking speed in the case the displacement speed specified by GOFR (EH 0.5) is higher than the actual displacement velocity and vice versa. Interceptive task Numerical simulations allow several predictions to be made for each strategy (Figure 43). These simulations were based on the average coefficients (k1 and k2, cf., Equations 1 and 2) found by Morice et al. (2010) and the bias in perceived velocity found by François et al. (under review). Following the results of Morice et al. (2010), the different display conditions should favor the use of a specific law of control. In the No-Path-Display condition the use of a CBA strategy should give rise to distinct displacement velocity profiles for the different curvature conditions. Moreover, Eye Height should influence the displacement velocity profiles moderately; more precisely manipulating eye height should influence essentially the initial conditions (i.e., the participant’s velocity when the trial begins). In the Path-Display condition the use of a MRV strategy should lead the participants to produce the same velocity profile whatever curvature condition. Conversely, manipulating Eye Height should give rise to clearly distinguishable velocity profiles with a very slow convergence of the curves as compared to the No-Path-Display condition. Finally, it is worth noting that the velocity adjustments resulting from the use of a CBA strategy should lead the participant to succeed in the task under all experimental conditions, while the operation of a MRV strategy should lead the participant to fail when Eye Height is increased or decreased, relative to normal, with final errors in the order of +/- 0.8 m. – 113 – Figure 43 : Numerical simulations of the walking speed provided by the CBA (A) and the MRV (B) models, as a function of ball path Curvature and Eye Height. RESULTS Walking task A three-way repeated measures ANOVA (3 Preparation Velocities × 3 Eye Heights × 8 Time Intervals) with displacement speed as dependent variable revealed significant main effects of Eye Height (F(2,14) = 109.55, P < 0.05, η²p = 0.94) and Time Intervals (F(7,49) = 12.60, P < 0.05, η²p = 0.64), but no significant effect of Preparation (F(2,14) = 0.30, P > 0.05, η²p = 0.04). A posteriori comparisons revealed that participants increased their walking velocity (Figure 44) when Eye Height was increased (EH 2), in comparison with the control condition (EH) (1.30 vs. 1.19 m/s, P < 0.05). Conversely, participants decreased their walking velocity in comparison with the control condition when Eye Height was decreased (EH 0.5) (0.93 vs. 1.19 m/s, P < 0.05). These results are in agreement with those obtained by François – 114 – et al. (under review); decreasing Eye Height gives rise to an overestimation of walking speed and as a consequence to a slowing down of locomotion pace (and vice versa). Moreover, the fact that velocity of walking during the preparation phase does not affect speed adjustments during the test phase led us to remove this factor from the remaining analyses. Figure 44 : Average walking velocity during the locomotion task of the test phase as a function of Eye Height conditions. Participants’ walking velocity was higher in EH 2 condition than in the control condition. Participants’ walking velocity was lower in EH 0.5 than in the control condition. The errors bars represent between-participant standard deviations. Interceptive task

Performance

Three-way repeated-measures ANOVAs (3 Eye Heights × 2 Curvatures × 2 Display Conditions) with Success Rate as dependent variable revealed a main significant effect of Display factor (F(1,7) = 15.06, P < 0.05, η²p = 0.69) (Figure 45A). A posteriori comparisons revealed that participants performed better in the Path-Display condition than in the No-Path- – 115 – Display condition (81.7 vs., 75.5 %, respectively). A three-way repeated-measures ANOVAs (3 Eye Heights × 2 Curvatures × 2 Display Conditions) with Constant Error as dependent variable revealed a main significant effect of Curvature (F(1,7) = 29.70, P < 0.05, η²p = 0.81) (Figure 45B). A posteriori comparisons revealed that participants arrived slightly early at the interception point (negative errors: -0.1 m) with negative curvature and slightly late (positive error: 0.18 m) with rectilinear trajectory. Figure 45 : Average success rate (A) and constant error (B) as a function of ball path display and ball path curvature. (A) Participants performed better in the Path-Display condition than in the No-Path-Display condition. (B) Participants reached the interception point (negative errors) with negative curvature slightly early and late (positive error) with rectilinear trajectory.

Kinematics

A four-way repeated-measures ANOVA (3 Eye Heights × 2 Curvatures × 2 Display conditions × 10 Time Intervals) with walking speed as dependent variable revealed significant main effects of Eye Height (F(2,14) = 69.67, P < 0.05, η²p = 0.91) and Curvature (F(1,7) = 46.65, P < 0.05, η²p = 0.87). We also found significant interactions between Eye Height and Time Intervals (F(18,126) = 27.92, P < 0.05, η²p = 0.79), Curvature and Time Intervals (F(9,63) = 116.58, P < 0.05, η²p = 0.94), Display and Time Intervals (F(9,63) = 22.66, P < 0.05, η²p = 0.76). and Eye Height, Curvature, Display and Time factors (F(18,126) = 2.14, P < 0.05, η²p = 0.23). Post-hoc analyses performed on this last interaction revealed several important effects. First of all, the time course of walking speed is affected differently by the curvature manipulations depending on the presence (or not) of ball-path display. In the No-Path-Display condition (left panels in Figure 46), the negative curvature condition gave rise to more pronounced changes in displacement speed than the rectilinear condition. More precisely, the negative curvature – 116 – conditions gave rise to a decrease in displacement velocity in the first part or the trial followed by a pronounced increase in displacement velocity in the second part of the trial (P<.05). Conversely, in the ball-path display condition, the reverse picture was observed. The displacement velocity changes were more pronounced in the rectilinear condition in comparison with those produced in the negative curvature condition. In this last condition, an increase in displacement velocity was observed in the first part of the trial, followed by a pronounced decrease in velocity during the second part of the trial (P<.05). Finally, the marked difference in initial displacement velocity in the three Eye Height, conditions whatever the experimental condition (i.e., Curvature and Display Conditions), indicated that we had succeeded in manipulating an optical correlate of displacement velocity. Interestingly a posteriori comparisons indicate a late convergence of the velocity profiles corresponding to the three Eye Height conditions in the Path-Display condition in comparison with the No-Path-Display condition (red zones in the Figure 46). While the velocity profiles can still be differentiated 3 seconds after the beginning of the trial in the Path-Display condition, the convergence appears earlier (after 2 seconds) in the No-Path-Display condition (P<.05).