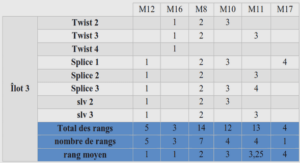
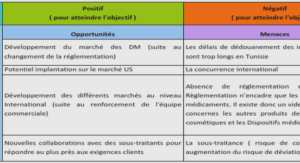
COLLECTIVE BLADE PITCH CONTROL OF FLOATING WIND TURBINES

The main objectives of controlling a traditional onshore wind turbine in Region III are to ensure a rated production of electrical power. In order to meet these objectives, many control strategies have been proposed (Menezes Novaes, Araújo, and Bouchonneau Da Silva 2018). However, these control algorithms can not be directly applied to the floating wind turbines due to the introduction of the floating platform: the dynamics of floating platform, particularly the platform pitch motion must Recalling the General Introduction, the main control objectives of FWT in Region III are to main- tains a rated power meanwhile reducing the platform pitch motion (J. Jonkman, Butterfield, et al. 2009). Many works have been done during the last decade on this problem. Linear control based on collective blade pitch (CBP) strategy (control of the three blades pitch angles by a single con- trol command) such as GSPI controller (J. Jonkman 2008a), linear quadratic regulator and linear parameter-varying controllers (Bagherieh and Nagamune 2015), model predictive control and feed- forward control (Schlipf, Pao, and Cheng 2012; Schlipf, Simley, et al. 2015). Most control approaches are based on linearized models of FWT (see previous chapter) that are derived from FAST software around an operating point depending especially on the wind and rotor speed. Consequently, the parameters of the controllers (that are mostly linear ones) must be tuned in different operating points to keep high performances; this tuning process has a cost and can be fastidious. A solution is the use of nonlinear control algorithms that have larger operating domains. In (Sandner et al. 2012; Schlipf, Sandner, et al. 2013; Raach et al. 2014; Homer and Nagamune 2018), nonlinear control strategies have been applied based on nonlinear models.

high order sliding mode control algorithms (Y. Shtessel et al. 2014; Cruz-Zavala and J. Moreno 2016) combined with gain/parameter adaptation laws (Yuri Shtessel, Taleb, and Plestan 2012; Tahoumi, Plestan, et al. 2019; S. Gutierrez et al. 2020) are well adapted. Such control algorithms are efficient even if the knowledge on the models is very limited and they are robust versus uncertainties and perturbations. In the sequel, the main contributions include Recalling that the FWT admits 4 operating regions (see Subsection Operating regions in General Introduction), this work is focused on the control problems in the Region III (also know as above- rated region). For the FWT system, the control problems in the considered region are firstly, the regulation of the power output at its rated value P , preventing an overload so as to protect the electric machine and the mechanical structure. Secondly, due to the additional DOFs introduced by the floating platform, the platform motion, especially the platform pitching, must be taken into consideration in order to avoid the negative damping (Skaare et al. 2007); as conclusion, the plat- form pitch motion must be reduced.

Existing solutions are, as mentioned in General Introduction, mainly based on the following ideas to solve this problem: the first solution is to use the detuned GSPI controller (Larsen and Hanson 2007; J. Jonkman 2008a) such that the natural frequency of closed-loop system is lower than the platform pitch natural frequency; this approach successfully attenuating the platform pitch motion but at a cost of larger power fluctuation. The second solution is based on modern control theory, such as LQR (Hazim Namik, Karl Stol, and J. a. Jonkman 2008; Christiansen, Knudsen, and T. Bak 2011; Christiansen, Knudsen, and T. Bak 2014) and H (Bakka and Karimi 2012; Bakka and Karimi 2012; X. Li and Gao 2015; Hara et al. 2017). The third solution is to use IBP control to increase the number of the inputs (Hazim Namik and Karl Stol 2010; H. Namik and K. Stol 2014; Lemmer, Raach, et al. 2015; Suemoto, Hara, and Konishi 2017); thus, multiple control objectives can be achieved. However, these solutions induce a great tuning effort due to the fact that the control is based on numerous linearized models, each model being obtained for an operating point. Moreover, the IBP control significantly increases the use of blade actuator comparing with the collective one, and is not completely implemented in commercial wind turbines (Menezes Novaes, Araújo, and Bouchonneau Da Silva 2018).