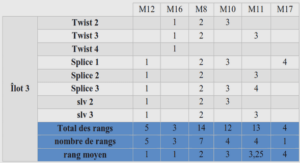
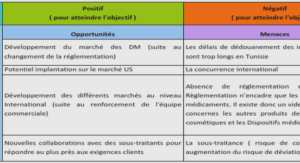
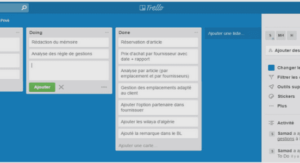
Cas de la réfléxion d’un choc oblique sur une couche limite laminaire

Dans ce chapitre nous étudierons la dynamique d’une réflexion de choc sur une couche limite laminaire. L’enssemble de ces travaux a fait l’objet d’une soumission pour publication dans « Journal of Fluid Mechanics » le 12 décembre 2017. Nous utiliserons, pour présenter ces résultats, l’article tel qu’il a été soumis. Nous passerons donc pour ce chapitre à la langue anglaise. Il est à noter que les annexes de cet article ont été déplacées à la fin du manuscrit. Shock wave/ boundary layer interactions (hereafter referred as SWBLI) play an importantrole in high-speed aerodynamics. Above critical conditions the interacting flow generally separates, with potential unsteady and three-dimensional evolutions (Délery et al.[35]). In case of an incoming laminar wall flow, of particular interest here, the interaction generally causes the transition to turbulence, possibly in the region of a laminar separation bubble (Schlichting [109]). Such laminar configurations are typical of flows through air intakes, past laminar wings and turbine blades for which the low turbulence level of the incoming flow allows laminar flow to develop on the fore part of the body. From an engineering point of view, all these phenomena imply significant deterioration of the aerodynamic loads and heat transfers compared to the baseline that challenges current engineering computational tools (Babinsky et al.[11] among others). One of the difficulties relates to the unsteady content of the flow.

In particular a low frequency unsteadiness of the shock in both turbulent and laminar SWBLIs has been repeatedly reported in the literature (for instance Dolling [36], Clemens et al.[25]) and fluctuations at well defined and higher frequencies are similarly observed, as The low frequency unsteadiness certainly represents the major concern as it may involve global flow oscillations in machines and thus deteriorate mechanical parts. In turbulent cases featuring flow separation at the shock foot, the low frequency component has first been analyzed as the response to fluctuations coming from the boundary layer upstream of the interaction. Ganapathisubramani et al.[43, 44] and Samimy et al. [102] related this low frequency unsteadiness to the long streamwise vortical structures peculiar of high Reynolds numbers turbulent boundary layers and in an experiment Unalmis and Dolling [121] found that Görtler vortices formed by the concavity of the nozzle present upstream of the interaction were involved. Other investigations associate this unsteadiness to a flapping of the bubble, see Dupont et al.[39], Pirozzoli et al.[96], Touber and Sandham [120] and Grilli et al.[52]. Carrying out Direct Numerical Simulations (DNS) of an oblique shock wave interaction with mean separation at M = 2.25, Pirozzoli et al.[96] observed a characteristic frequency of S≃ 0.03 and suggested that this unsteadiness could be driven by a resonance mechanism involved by the combination of the propagation of large vortical structures created in the shear layer of the fore part of the bubble with a feedback caused by pressure disturbances traveling backward in the slow region inside the bubble. In this scenario the impact of the vortices upon the shock causes the oscillation of the reflected shock wave which is present to accommodate the orientation of the flow about the wall. This analysis implies that correlations between the low frequency motion of the reflected shock and the fluctuations of the bubble must be present and these were indeed observed by Thomas et al.[118] for the flow over a compression ramp between the reflected shock and the reattachment region.

The low frequency unsteadiness is also observed in transitional configurations, for which the flow upstream of the interaction is laminar and becomes turbulent downstream of the interaction. Sansica et al.[103], through a DNS analysis of a two-dimensional (2D) interaction at M = 1.5 forced either upstream of the separation, inside the circulation bubble or both, found this low frequency unsteadiness to be particularly strong near the separation point and present even in the absence of an upstream forcing. This indicates that incoming perturbations are not necessary to promote the low frequency unsteadiness in this type of interactions. Sansica et al.[104] further analyzed this question by combining DNS simulations with local and parabolized stability analysis. The result is that the flow in the interaction is convectively unstable due to oblique mode growth (on this subject, see also the work of Chang et al [23]) and that the bubble acts like a filter-amplifier of low frequency disturbances at about S ∼ 0.1. In addition the DNS simulation shows the existence of a broad band spectrum of low frequency fluctuations localized in the vicinity of the separation point. The broad band is centered around a Strouhal number of S.