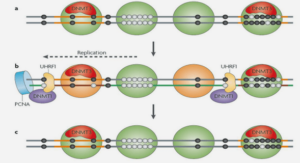
Emergent Geometry and Gauge Theory in 4 Dimensions and The Noncommutative Torus

Emergent Geometry and Gauge Theory in 4 Dimensions and The Noncommutative Torus

 Noncommutative gauge theory

Star-gauge invariant action To define a YangMills theory on a noncommutative plane we have to generalize the map (2.62). Let Aµ(x) be a Hermitian gauge field on R d , which corresponds to the unitary gauge group U(n). We can introduce the Weyl operators corresponding to Aµ(x) by taking the trace of the tensor product of ∆(x) and the gauge field [50] Aˆ µ = Z d dx∆(x) ⊗ Aµ(x) (2.78) the derivative of Weyl operators is equal to the Weyl operator of the usual derivative of the functions [50] [ ˆ∂µ, W[f]] = Z d dx∂µf(x)∆(x) = W[∂µf] (2.79) Based on this equation a noncommutative version of the Yang-Mills action can ce defined S[Aˆ] = − 1 4g 2 Tr trN   ˆ∂µ, Aˆ ν  −  ˆ∂ν, Aˆ µ  − i  Aˆ µ, Aˆ ν  2 (2.80) where the term in brackets is the operator analog of the field strength tensor. Here Tr is the operator is given by an integration over space-time Tr W[f] = Z d dx f(x) (2.81) and trN denotes the trace in color space. This action is invariant under transformations of the form Aˆ µ → UˆAˆ µUˆ† − iUˆ  ˆ∂µ, Uˆ  , (2.82) where Uˆ as an arbitrary unitary element of the algebra of matrix valued operators, i. e. UˆUˆ† = Uˆ†Uˆ = 1ˆ ⊗ 1n (2.83) The symbol 1ˆ is here the identity on the ordinary Weyl algebra and 1n is a n × n unit matrix.  To set up the action in coordinate space we can construct an inverse map of (2.78). The Yang-Mills action in coordinate space the reads S[A] = − 1 4g 2 Z d dxtrN .

Photon Self-energy and UV/IR

Mixing Now, using the vertex function (2.106), we find the first order fermion loop correction to the photon propagator given by the one-loop photon selfenergy diagram in Fig. (4.2) to be Π µν (1)(k) = −4 Z d 4p (2π) 4 ×  T µν + i 2 sim( 1 4 p ∧ k) 1 4 p ∧ k  (˜p − 1 2 ˜k) µ kρT ρνe − i 4 p∧k − (˜p − 1 2 ˜k) ν kρT ρµe i 4 p∧k  + i 4 sim2 ( 1 4 p ∧ k) ( 1 4 p ∧ k) 2 (˜p − 1 2 ˜k) µ (˜p − 1 2 ˜k) ν kρkσT ρσ (2.107) where T µν(k, p) := (p − k) µp ν + p µ (p − k) ν + [m2 − (p − k).p]η µν [(p − k) 2 − m2 ][p 2 − m2 ] , (2.108) which is the only term we get in the commutative case. Therefore, the first term in (2.107) is naturally understood to correspond to the planar part of the diagram, and in fact follows straightforwardly from the first terms of the vertex functions (2.106) as the phase factors cancel each other, in the same way as they do for the planar diagrams of a noncommutative scalar field theory. The other terms, on the other hand, clearly correspond to the nonplanar part with nontrivial phase factors that give rise to UV/IR mixing. Indeed, the second term in (2.106) can be shown to yield the leading order contribution (The third term leads also to similar IR-divergent terms) iΠ µν (1)np (k) ≈ 8 π 2 ˜k µ˜k ν ˜k 4 4 π 2 ˜˜k µk ν + k µ˜˜k ν ˜k 4 (2.109) at the IR-limit of the external momentum. The first term in (2.109) is similar to (2.98) found in the naive formulation above, whereas the second term is gauge variant and should cancel, when all the second order contributions in the coupling constant are taken into account. Therefore we conclude that also gauge field theories defined via Seiberg − W itten map appear to fail to be renormalizable because of UV/IR mixing, which further shows that this is a generic property of noncommutative theories.

 Reduced Models and Emergent Phenomena

In this part we will work out the nonperturbative, construction definition of noncommutative Yang-Mills theory. this can be completely described in the language of matrix models (arising here as reduced models). This will also reveal some beautiful features of the vacuum structure of noncommutative gauge theories. Will remove derivative operators ∂i or ˆ∂i from the noncommutative gauge theory action. There is no analog of this manipulation in ordinary Yang-Mills theory [53]. Let us introduce covariant coordinates: Cˆ i = (θ −1 )ij ˆx j + Aˆ i (2.110) Then Cˆ i → UˆCˆ iUˆ† under gauge transformations. We can be represented the adjoint actions (θ −1 )ij [ˆx j , −] (2.111) i.e. ˆ∂i are inner derivations of the algebra Rd θ . Then the entire noncommutative gauge theory can be rewritten in terms of the Cˆ i , we may rewrite the covariant derivative as: ∇ˆ i = ˆ∂ 0 i − iCˆ i (2.112) where ˆ∂ 0 i = ˆ∂i + i(θ −1 )ijxˆ j . Then using [ ˆ∂ 0 i , xˆ j ] = 0, We compute: [∇ˆ i , ˆf] = −i[Cˆ i , ˆf] (2.113) Fˆ ij = i[∇ˆ i , ∇ˆ j ] = −i[Cˆ i , Cˆ j ] + (θ −1 )ij (2.114) and consequently, SY M = 1 4g 2 TrX i6=j