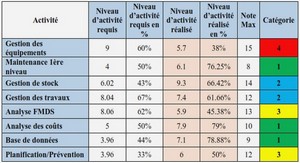
Analysis of ceramic foams

Analysis of ceramic foams

X-ray Computed Tomography (CT) 

It is necessary to precisely determine the foam’s geometry to predict combustion processes in the medium and to develop numerical models based on experimental data. The structural irregularities of ceramic foams make them difficult to reconstruct with conventional computer aided design (CAD) tools. Thus, using an approach that allows us to obtain accurate foam geometry is necessary to achieve accurate results. For this task, we chose Xray Computed Tomography (CT), representing a non destructive form of geometric analysis. A CT scan takes a series of X-ray measurements of a given object under various angles and combines the results into cross-sectional images (an image stack) with the further possibility of recreating the object’s volume (S. L. Wellington & H. J. Vinegar, 1987).

The main steps undertaken during CT scan of foam specimens are described below:
1) The specimen was installed into Nikon XT H 225 X-ray μ-CT system (Nikon, Brighton, MI, USA). Data was obtained with 220 kV tube voltage and 350 µA current, and complemented with a 1 mm-thick copper filter.
2) Obtained volume was reconstructed using the CT Pro 3D software (Nikon, Brighton, MI, USA) and a stack of image slices was obtained for each foam.
3) The image stack was imported afterwards into VG Studio MAX 3.0 (VG) software (GmbH Volume Graphics, 2016). Surface, based on the greyscale threshold, was determined and the volume was recreated.

VGStudio Max 3.0 (3D analysis)
To evaluate the porosity of foams with high PPI, for which the application of Archimedes’ principle failed, VGStudio Max 3.0 software (VG) was employed to analyze the reconstructed volumes from the CT scan data. During reconstruction, there were two main parameters that influenced the final 3D model: “Pixel resolution” and “grey threshold” value. Pixel resolution is a scaling factor, defined during the generation of the image stack or calculated from a cross-section image as the ratio of known physical length to its length in pixels. Each pixel of the greyscale image has its value, and by applying a given grey threshold, the pixel’s domain becomes separated on the void and solid phases. Application of “pixel resolution” and “grey threshold” values result in volume recreation with correct overall dimensions and creates a correctly reconstructed shape.

Watershed method (2D analysis)
One approach used to determine a foam’s cell size, which is widely used, is watershed segmentation (A. P. Mangan & R. T. Whitaker, 1999). This method uses the analogy of a geological watershed and applies it to foam, transforming an image into a topographic map with corresponding basins and peaks . The initial image  is transformed into a map  that represents the distance from each void pixel (white) to the nearest material pixel (black), and the obtained distances are interpreted as geological depth. Afterwards, the pixels are filled from most to least deep. When corresponding “flows” from various basins meet, dividing watershed lines are created, forming segmented cells  . The initial material is then added, and the area of each cell ( Ac ) is calculated  . By using the described principle, we applied watershed segmentation for each image stack by writing a MATLAB script.

VGStudio Max 3.0: Watershed method (3D analysis)
The “Foam Structure Analysis” module of the VG software uses a more sophisticated approach to provide 3D watershed segmentation (A. Videla et al., 2006). This is similar to the previously described technique applied for 2D images, where pixels are replaced by their 3D volumetric analogues, voxels. VG creates a distance map from each void voxel (the 3D equivalent of a 2D pixel) to the nearest material voxel. The farther a void voxel is located, the deeper it lies within the “watershed.” Like the method for 2D segmentation, void voxels are filled from most to least deep, until the corresponding cell volumes meet and watershed  segmentation occurs. The software visualizes   and provides detailed information about each cell that is found (volume, surface area, etc.).

VGStudio Max 3.0: Maximal inscribed spheres method
The “Capillary Pressure Curve” module in VG allows for the computation of a mean cell diameter (݀dcsph), using the approach of maximal inscribed spheres. This approach uses spheres of various sizes as probes and inscribes them into the void space between struts until the corresponding maximal dimension is found.